Math doesn’t change depending on country
PEMDAS and BEDMAS are just English acronyms which means you might’ve gotten something else to memorize it
umm so what does it stand for maybe i have a different series of letters
PEMDAS is
Parenthesis - Exponents - Multiplication/Division - Addition/Subtraction
BEDMAS is
Brackets - Exponents - Division/Multiplication - Addition/Subtraction
oh shit i forgot about that
lol i am too bad at math for this
i got 3
what if you get it wrong just for the lulz
I admit I did it twice, first got 1 then 9. Which is surprising, considering I make some mistakes at maths but this was pretty easy.
This is what I had used to get one.
6 ÷ 2(1+2) → 6 ÷ 2(3) → 6 ÷ 6 → 1
At the end of the day, this doesn’t even really matter. It’s just a matter of notation.
If you are of the belief that 6 ÷ 2(1+2) is equivalent to (6 ÷ 2)(1+2), then you get 9. If you believe it is equivalent to 6 ÷ (2(1+2)), you get one.
In my own calculation, the reason why 6 ÷ 2(3) simplifies to 6 ÷ 6 is that I was either taught or got the idea that an expression of the form x(y) was “bracketed”, and therefore took priority over everything else.
In fact, I’d say the sheer fact that so many people are getting it wrong and calculating it in this exact alternate way shows that it isn’t just people getting their algebra wrong here. It’s probably something else. Alternate teaching methods, perhaps? I don’t care enough to start digging into it.
This isn’t a problem with Mathematics as a concept so much as it is notation, to be honest.
Perfectly conceivable that such a thing could happen. Especially seeing as these kinds of expressions can be easily disambiguated by brackets/parentheses. It’s not like they’re saying 1 + 1 = 5.
Notation is the same for most countries, naming convention might be different but the notation itself remains the same
Damnit I just realized how its nine
Wait a minute no I was right start with brackets
I just distributed before I multiplied anything.
1+2=3 6/2=3
3x3=9
I can see how it’s both 1 and 9, but I got 1 when I first did it.
When you do:
6 / 2(1+2) =
6 / 2(3) =
6 / 6 = you will get 1
But when you do
6 / 2(1+2) =
3(1+2) =
3(3) = You get 9.
I believe 1 is the correct answer tho
Whoever said it isn’t 1 is uh. They should redo their math.
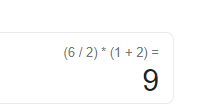
ok
That the calculator working from left to right and not following the order of operations.
what